meter per second (m/s)
What is meter per second (m/s)?
The meter per second (m/s) is the standard unit of speed and velocity in the International System of Units. Speed is a scalar quantity that indicates the magnitude, or rate, of an object's movement, as determined by the amount of distance the object travels over a specific time interval. Velocity is a vector quantity that indicates both the magnitude and direction of an object's movement. It is concerned with the rate at which the object's position changes over a time interval.
A speed or velocity measurement in m/s is considered either average or instantaneous. An object's average speed takes into account distance and time, whereas the average velocity takes into account the object's displacement -- or change in position -- and time. Calculating average speed or velocity is simply a matter of dividing the distance or displacement by the time interval. This is explained more in the following sections.
Instantaneous speed and velocity are more complex. Similar to average speed and velocity, instantaneous speed focuses on distance over time, and instantaneous velocity focuses on displacement over time. An instantaneous measurement attempts to calculate the distance or displacement at the smallest possible time interval that the measuring technique allows. The interval should be as close to zero as possible to approximate an instant of time. For example, a car's speedometer shows its instantaneous speed at a given moment, as does a state trooper's radar gun.
Calculating an instantaneous speed or velocity requires the use of a mathematical limit formula that specifies time approaching zero. A limit formula is used in calculus to determine the derivative of a function -- in this case, distance or displacement as it relates to time. Because of the complexity involved, calculating instantaneous speeds or velocities is beyond the scope of this definition.
Calculating average speeds
You can calculate an object's average speed by dividing the distance traveled by the time it takes the object to travel that distance. Many different metrics are used to measure speed, such as kilometers (km) per hour (kph), miles per hour, feet per second or meters per second. When m/s is used, the distance traveled is measured in meters (m), and the interval of time is measured in seconds (s).
The following formula can be used to calculate an object's average speed:
savg = d / t
The savg represents the average speed that the object traveled. It is equal to the distance (d) divided by the amount of time (t) it took the object to travel that distance. Note that the letters and symbols used in the speed and velocity formulas in this article often vary from one source to the next.
To understand how the speed formula works, consider a car that has traveled 250 meters in 10 seconds. From this information, you can use the formula to determine the car's average speed in meters per second:
savg = d / t
savg = 250 m / 10 s
savg = 25 m/s
The average speed during the 10-second interval was 25 m/s. If the car had traveled at the same rate for one hour, its average speed would have been 90 kph, which can be verified with the following calculations:
savg = d / t
savg = 90 km / 1 h
savg = 90,000 m / 3,600 s
savg = 25 m/s
Calculating average velocities
The previous calculations demonstrated that average speed is a measurement of distance over time. Finding an object's average velocity requires a different formula because it is concerned with the rate of displacement rather than the distance traveled.
Displacement in this sense refers to the change in position from a starting point or initial position to an ending point or final position. It does not matter what route the object traveled between the two points. It is concerned only with the difference between these positions.
To calculate an object's velocity, divide the displacement by the amount of time it took to move from the initial position to the final position, as shown in the following formula:
vavg = ∆x / ∆t
The vavg represents the object's average velocity. The Greek letter delta (∆) means "change in" the variable that follows the delta. In this case, ∆x indicates the change in position, or displacement, and ∆t indicates the change in time. The ∆x and ∆t can also be represented by the following formula:
vavg = (xf − xi) / (tf − ti)
The xf variable is the final position, xi is the initial position, tf is the final time, and ti is the initial time. For example, consider a car that travels west along a 1,000-meter stretch of highway. When the car reaches the 750 m mark, a timer starts. When the car reaches the 1,000 m mark, the timer stops, showing that a 10-second interval has passed. From this information, you can calculate the car's average velocity:
vavg = ∆x / ∆t
vavg = (xf − xi) / (tf − ti)
vavg = (1,000 m − 750 m) / (10 s − 0 s)
vavg = 250 m / 10 s
vavg = 25 m/s
The car's average velocity was 25 m/s during the timed interval. This is the same amount as the car's average speed shown in the previous example. Based on these results, it might appear that speed and velocity are the same thing. However, speed is concerned with distance, whereas velocity is concerned with displacement, which can make a significant difference in the final results.
Average speed vs. average velocity
Distance cares about the route taken to get from point A to point B. Displacement does not. The example in the following figure helps to illustrate this point.
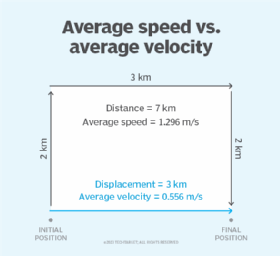
The figure shows the path taken by two people who walked for 90 minutes. They headed north for 2 km, then east for 3 km and finally south for 2 km, resulting in a total of 7 km.
Although the two people walked 7 km, the difference between the initial position and final position is only 3 km. This is the displacement. It is not concerned with what route they took or whether they backtracked or even walked in circles. It is concerned only with their starting and ending positions, along with the time it took to get from one to the other.
With this in mind, you can calculate the walkers' average speed and velocity by using the same formulas as above, starting with the one for speed:
savg = d / t
savg = 7 km / 90 min
savg = 7,000 m / 5,400 s
savg = 1.296 m/s (approximate)
The results indicate that their average speed was about 1.296 m/s. However, as the following calculations show, the average velocity is much lower, only 0.556 m/s:
vavg = ∆x / ∆t
vavg = (xf − xi) / (tf − ti)
vavg = (3 km − 0 km) / (90 min − 0 min)
vavg = 3 km / 90 min
vavg = 3,000 m / 5,400 s
vavg = 0.556 m/s (approximate)
The calculations are based on the assumption that the initial position is 0 km and the initial time is 0 s, with the final position 3 km east of the original position.
What is kilo, mega, giga, tera, peta, exa, zetta and all that?
